The centre of
mass or mass centre is
the mean location of all the mass
in a system. In the case
of a rigid body,
the position of the centre of mass is fixed in relation to the body. In the
case of a loose distribution of masses in free space,
such as shot
from a shotgun
or the planets
of the solar system,
the position of the centre of mass is a point in space among them that may
not correspond to the position of any individual mass.
The term centre of mass is often used interchangeably with centre
of gravity, but they are physically different concepts. They
happen to coincide in a uniform gravitational field, but where gravity is not uniform, centre of
gravity refers to the mean location
of the gravitational force acting on a body.
The centre of mass of a body does not generally coincide with its
geometric centre, and this property can be exploited. Engineers try to design a
sports car‘s
centre of mass as low as possible to make the car handle
better. When high jumpers
perform a “Finsbury Flop“,
they bend their body in such a way that it is possible for the jumper to clear
the bar while his or her centre of mass does not.
CENTRE OF MASS OF A REGULAR OBJECT
If an object has uniform density
then its centre of mass is the same as the centroid
of its shape.
Examples:
·
The centre of mass of a ring is at the centre of the
ring (in the air).
·
The centre of mass of a solid triangle lies on all
three medians and therefore at the centroid,
which is also the average of the three vertices.
·
The centre of mass of a rectangle is at the
intersection of the two diagonals.
·
In a spherically symmetric body, the centre of mass is
at the centre. This approximately applies to the Earth: the density varies
considerably, but it mainly depends on depth and less on the latitude
and longitude
coordinates.
·
More generally, for any symmetry of a body, its centre
of mass will be a fixed point of that symmetry.
CENTRE OF
MASS OF AN ARBITRARY 2D PHYSICAL SHAPE
The Centre of
Mass of an arbitrary 2D physical shape can be found using the following
plumbline method as illustrated in the following table:
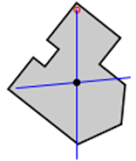
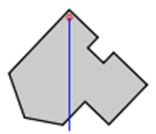
|
||
|
Step 1: An arbitrary 2D shape.
|
Step 2: Suspend the shape from a location near an edge. |
Step 3: Suspend the shape from another location not too |
Table 4.2: Centre of
Mass of an arbitrary 2D physical shape
This method is useful when one wishes to find the centroid
of a complex planar shape with unknown dimensions. It relies on finding the
centre of mass of a thin body of homogenous density
having the same shape as the complex planar shape.
CENTRE OF
MASS OF AN L-SHAPED OBJECT
This is a method of determining the centre of mass of an L-shaped
object.
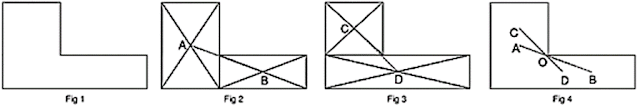 |
| Centre of Mass of an L-shaped object |
1.
Divide the
shape into two rectangles, as shown in fig 2. Find the centre of masses of
these two rectangles by drawing the diagonals. Draw a line joining the centres
of mass. The centre of mass of the shape must lie on this line AB.
2. Divide the shape into two other rectangles, as shown
in fig 3. Find the centres of mass of these two rectangles by drawing the
diagonals. Draw a line joining the centres of mass. The centre of mass of the
L-shape must lie on this line CD.
3. As the centre of mass of the shape must lie along AB
and also along CD, it is obvious that it is at the intersection of these two
lines; at O. (The point O may or may not lie inside the L-shaped object.)
Discover more from Umuco Nyarwanda
Subscribe to get the latest posts sent to your email.